Answer:
25.28 cm
Explanation:
The chord is 6cm away from the centre of the circle . And the radius of the circle is 14 cm . We know that the perpendicular from the centre bisects the chord .
This will form a right angle triangle with the distance between centre and the code as its perpendicular and the length of radius as hypotenuse .
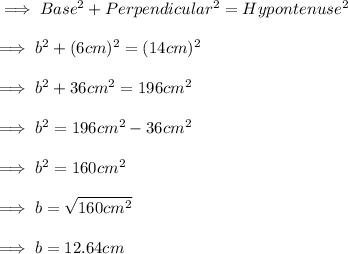
Hence the required answer is 12.64 × 2 = 25.28cm .