Question

Solve for the missing sidebof the triangle shown in the figure​

Answer 1

∠B = 74°

a ≈ 14.5 inches

c ≈ 11.4 inches

Explanation:

The given parameters of triangle ΔABC are;

∠A = 62.2°, ∠C = 43.8° and side
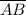`\overline{AB}` = b = 15.8 in

By sine rule, we have;

`(a)/(sin(\angle A)) = (b)/(sin(\angle B)) = (c)/(sin(\angle C))`

`a = {sin(\angle A)} * (b)/(sin(\angle B))`

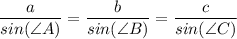
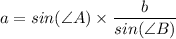
By angle sum property, we have;

∠B = 180° - (∠A + ∠C)

∴ ∠B = 180° - (62.2° + 43.8°) = 74°

∠B = 74°

`\therefore {a} = {sin(62.2^(\circ))} * (15.8)/(sin(74^(\circ))) \approx 14.5`

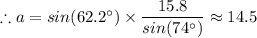
a ≈ 14.5 in.

`c = {sin(\angle C)} * (b)/(sin(\angle B))`

`\therefore {c} = {sin(43.8^(\circ))} * (15.8)/(sin(74^(\circ))) \approx 11.4`

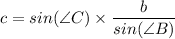
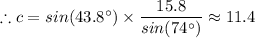
c ≈ 11.4 in.