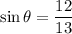Question

The terminal side of 0 is in quadrant II and cos 0 = -5/13. What is sin 0?

Answer 1

What the person above me said love
Hope it helps!

Answer 2

`\displaystyle \sin\theta=(12)/(13)`

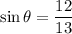
Explanation:

We are given that:

`\displaystyle \cos\theta =-(5)/(13)\text{ where $\theta$ is in QII}`

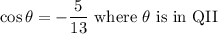
Recall that cosine is the ratio of the adjacent side to the hypotenuse. Using the Pythagorean Theorem, solve for the opposite side (we can ignore the negative for now):

`o=√(13^2-5^2)=12`

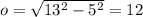
And since θ is in QII, sine is positive, and cosine and tangent are both negative.

Sine is the ratio of the opposite side to the hypotenuse. Therefore:

`\displaystyle \sin\theta=(12)/(13)`