Before we find the perimeter, first we must know length and width.
Let width = x
The question says that the length of a rectangle is twice its width. Therefore the length is 2×x or two times of width which equal to 2x.
The area of rectangle is given as 32 cm^2.
Do you remember the rectangle area formula? If not then it is:
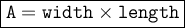
Since A is area - substitute A = 32 in. As for width, we substitute in x and length in 2x.
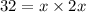
Since x multiplies by another x. It becomes x squared.
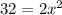
Next, we divide 2 by both sides since we want to solve for x.
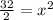
Simplify 32/2 in simplest form.
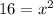
Square both sides so we can get rid the x squared, leaving only x.
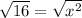
Normally, when solving quadratic equation, you must write plus or minus. However, for this circumstance - we are solving a variable for length and width which cannot be negative. Therefore
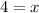
After we solve for x. Substitute x = 4 in width = x and length = 2x.
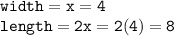
When we multiply these two numbers, we get 32 cm^2.
Next, we find the perimeter. The formula of perimeter for rectangle is:

We know width and length which are 4 and 8 in order. Substitute in the formula.
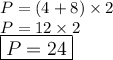
Hence, the perimeter is 24.
Answer
- The perimeter for the rectangle is 24.