Question

A 92-kg man climbs into a car with worn out shock absorbers, and this causes the car to drop down 4.5 cm. As he drives along he hits a bump, which starts the car oscillating at an angular frequency of 4.52 rad/s. What is the mass of the car ?A) 890 kg

B) 1900 kg
C) 920 kg
D) 990 kg
E) 760 kg

Answer 1

the mass of the car is 890 kg

Step-by-step explanation:

Given;

mass of the man, m = 92 kg

displacement of the car's spring, x = 4.5 cm = 0.045 m

acceleration due to gravity, g = 9.8 m/s²

The spring constant of the car,

f = kx

where;

f is the weight of the man on the car = mg

mg = kx

k = mg/x

k = (92 x 9.8) / 0.045

k = 20,035.56 N/m

The angular speed of car, ω, when the is inside is given as 4.52 rad/s

The total mass of the car and the man is calculated as;

`\omega = \sqrt{(k)/(m) } \\\\\omega^2 = (k)/(m) \\\\m = (k)/(\omega^2) = (20,035.56)/((4.52)^2) = 980.7 \ kg`

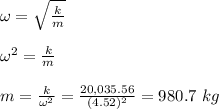
The mass of the car alone = 980.7 kg - 92 kg

= 888.7 kg

≅ 890 kg

Therefore, the mass of the car is 890 kg