Answer:
(a) t = 1.14 s
(b) h = 0.82 m
(c) vf = 7.17 m/s
Step-by-step explanation:
(b)
Considering the upward motion, we apply the third equation of motion:
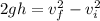
where,
g = - 9.8 m/s² (-ve sign for upward motion)
h = max height reached = ?
vf = final speed = 0 m/s
vi = initial speed = 4 m/s
Therefore,
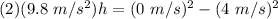
h = 0.82 m
Now, for the time in air during upward motion we use first equation of motion:
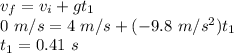
(c)
Now we will consider the downward motion and use the third equation of motion:
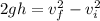
where,
h = total height = 0.82 m + 1.8 m = 2.62 m
vi = initial speed = 0 m/s
g = 9.8 m/s²
vf = final speed = ?
Therefore,
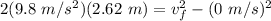
vf = 7.17 m/s
Now, for the time in air during downward motion we use the first equation of motion:
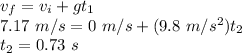
(a)
Total Time of Flight = t = t₁ + t₂
t = 0.41 s + 0.73 s
t = 1.14 s