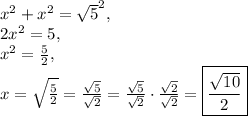Question

The figure below is a square. Find the length of side x in simplest radical form with a rational denominator.

Answer 1

`(√(10))/(2)`

Explanation:

The diagonal forms two 45-45-90 triangles, with the diagonal being the hypotenuse of both. The Pythagorean Theorem states that
`a^2+b^2=c^2`, where
`c` is the hypotenuse of the triangle, and
`a` and
`b` are the two legs of the triangle.

From the Isosceles Base Theorem, the two legs of a 45-45-90 triangle are always equal. Since we're given a diagonal of
`√(5)`, we have:

`x^2+x^2=√(5)^2,\\2x^2=5,\\x^2=(5)/(2),\\x=\sqrt{(5)/(2)}=(√(5))/(√(2))=(√(5))/(√(2))\cdot (√(2))/(√(2))=\boxed{(√(10))/(2)}`