Answer:
The margin of error is of 0.9089 hours.
Explanation:
We have the standard deviation for the sample, which means that the t-distribution is used to solve this question.
The first step to solve this problem is finding how many degrees of freedom, we have. This is the sample size subtracted by 1. So
df = 20 - 1 = 19
95% confidence interval
Now, we have to find a value of T, which is found looking at the t table, with 19 degrees of freedom(y-axis) and a confidence level of
 . So we have T = 2.093
. So we have T = 2.093
The margin of error is:
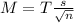
In which s is the standard deviation of the sample and n is the size of the sample.
20 students:
This means that

Standard deviation of 1.942.
This means that
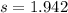
When estimating the average amount of sleep with a 95% confidence interval, what is the margin of error?
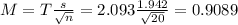
The margin of error is of 0.9089 hours.