Question

Find two numbers whose difference is 5 and sun of whose squares is 100.

(I believe this is the quadratic formula!)

I’m not sure how to go about it. If someone go go through it step by step, that’d be helpful. (Or even give me the values to put into the quadratic formula)

Answer 1

I. x = 14.115 and y = 9.115

II. x = 0.885 and y = -4.115

Explanation:

• Let the two numbers be x and y respectively.

Translating the word problem, we have;

x - y = 5 ......equation 1

x² + y² = 100 ...... equation 2

x = y + 5 ...... equation 3

Substituting eqn 3 into eqn 2, we have;

(y + 5)² + y² = 100

Simplifying further by opening the bracket, we have;

(y + 5)(y + 5) + y² = 100

y² + 5y + 5y + 25 + y² = 100

y² + 10y + 25 + y² = 100

2y² + 10y + 25 = 100

2y² + 10y + 25 - 100 = 0

2y² + 10y - 75 = 0

To find the roots of the quadratic equation, we would use the quadratic formula;

Note: the standard form of a quadratic equation is ax² + bx + c = 0

a = 2, b = 10 and c = -75

The quadratic equation formula is;

`x = \frac {-b \; \pm \sqrt {b^(2) - 4ac}}{2a}`

Substituting into the formula, we have;

`y = \frac {-10 \; \pm \sqrt {10^(2) - 4*2*(-75)}}{2*2}`

`y = \frac {-10 \pm \sqrt {100 - (-600)}}{4}`

`y = \frac {10 \pm \sqrt {100 + 600}}{4}`

`y = \frac {10 \pm \sqrt {700}}{4}`

`y = \frac {10 \pm 26.46}{4}`

`y_(1) = \frac {10 + 26.46}{4}`

`y_(1) = \frac {36.46}{4}`

`y_(1) = 9.115`

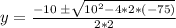
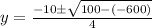
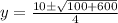
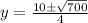
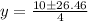
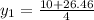
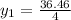
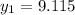
Or

`y_(2) = \frac {10 - 26.46}{4}`

`y_(2) = \frac {-16.46}{4}`

`y_(2) = -4.115`

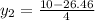
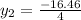
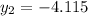
Next, we would find the value of x;

x = y + 5

When y = 9.115

x = 9.115 + 5

x = 14.115

When y = -4.115

x = -4.115 + 5

x = 0.885

Check:

x - y = 5

14.115 - 9.115 = 5