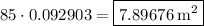Question

find the area of the following shapes. Show the formula used and all work. Round to 1 decimal place .

Answer 1

`\text{d. }106,250\:\mathrm{cm^2},\\\text{e. }38.5\:\mathrm{cm^2},\\\text{a. }85\:\mathrm{ft^2},\\\text{b. }7.89676\:\mathrm{m^2}`

Explanation:

Part D:

The figure shows a parallelogram with base 425 cm and height 250 cm. Its area can be found by
`A=bh` and therefore the area of this shape is
`A=425\cdot 250=\boxed{106,250\:\mathrm{cm^2}}`

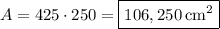
Part E:

The figure shows a trapezoid. The area of a trapezoid is equal to the average of its bases multiplied by the height. Since one base is 2 cm and the other base is 9 cm, the average of these bases is
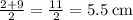`(2+9)/(2)=(11)/(2)=5.5\:\mathrm{cm}`. The height is given as 7 cm, therefore the area of the trapezoid is
`7\cdot 5.5=\boxed{38.5\:\mathrm{cm^2}}`

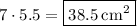
Part A:

The composite figure consists of two rectangles. The area of a rectangle with base
`b` and height
`h` is given by
`A=bh`. The total area of the figure is equal to the sum of the areas of these two rectangles.

Area of first rectangle (rectangle on bottom):
`5\cdot 13=65\:\mathrm{ft^2}`

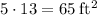
Area of second rectangle (rectangle on top):

*Since we don't know the dimensions, we must find them. Start by converting 108 inches to feet:

`108\:\mathrm{in}=9\:\mathrm{ft}`. Therefore, the dimensions of this rectangle are (10-5) ft by (13-9) ft
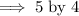`\implies5\text{ by } 4` and this rectangle's area is
`5\cdot 4=20\:\mathrm{ft^2}`

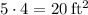
Thus, the area of the figure is equal to
`65+20=\boxed{85\:\mathrm{ft^2}}`

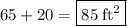
Part B:

We've already found the area of the figure in the previous part in square feet. To find the area in square meters, use the conversion
`1\text{ square foot}=0.092903\text{ square meter}`.

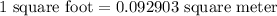Therefore, the area of the figure, in square meters, is
`85\cdot 0.092903=\boxed{7.89676\:\mathrm{m^2}}`