Question

What is the equation of the line that
contains the points (0, 5) and (3, 7)?

Answer 1

To find an equation of a line, you have to know the value of slope first. We can find the value of slope by using the formula below:

`\large \boxed{m = (y_2 - y_1)/(x_2 - x_1) }`

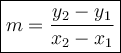
m-term represents the slope from y = mx+b.

We are given two coordinate points. Substitute the points in the formula.

`\large{m = (7 - 5)/(3 - 0) } \\ \large{m = (2)/(3) }`

Therefore the slope is 2/3.

Next we find the y-intercept. In the form of y = mx+b where m = slope and b = y-intercept. We have got slope, except the y-intercept. We can find the y-intercept by substituting one of gjven points in the equation of y = mx+b.

Since we know the slope - we can rewrite the equation like below:

`\large{y = (2)/(3) x + b}`

Then choose one of two points to substitute. I will choose (0,5). Therefore substitute x = 0 and y = 5.

`\large{5 = (2)/(3) (0) + b} \\ \large{5 = 0 + b} \\ \large{b = 5}`

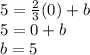
Thus the y-intercept is (0,5). Note that if the question gives you (0,a) point. That is the y-intercept of graph. So b = a if given (0,a).

Rewrite the equation by substituting the b-value.

`\large{y = (2)/(3) x + 5}`

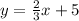
Hence, the equation of a line that contains those points is y = 2x/3 + 5

Answer

• y = 2x/3 + 5

Hope this helps! Let me know if you have any doubts.