Question

NASA launches a rocket at t = 0 seconds. Its height, in meters above sea-level, as a function of time is given by h ( t ) = − 4.9 t 2 + 229 t + 346 . Assuming that the rocket will splash down into the ocean, at what time does splashdown occur?

How high above sea-level does the rocket get at its peak?

Answer 1

`\displaystyle 1)48.2 \: \: \text{sec}`

`\rm \displaystyle 2)3021.6 \: m`

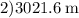
Explanation:

Question-1:

so when flash down occurs the rocket will be in the ground in other words the elevation(height) from ground level will be 0 therefore,

to figure out the time of flash down we can set h(t) to 0 by doing so we obtain:

`\displaystyle - 4.9 {t}^(2) + 229t + 346 = 0`

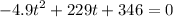
to solve the equation can consider the quadratic formula given by

`\displaystyle x = \frac{ - b \pm \sqrt{ {b}^(2) - 4 ac} }{2a}`

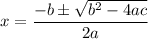
so let our a,b and c be -4.9,229 and 346 Thus substitute:

`\rm\displaystyle t = \frac{ - (229) \pm \sqrt{ {229}^(2) - 4.( - 4.9)(346)} }{2.( - 4.9)}`

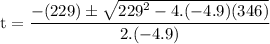
remove parentheses:

`\rm\displaystyle t = \frac{ - 229 \pm \sqrt{ {229}^(2) - 4.( - 4.9)(346)} }{2.( - 4.9)}`

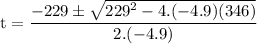
simplify square:

`\rm\displaystyle t = ( - 229 \pm √( 52441- 4( - 4.9)(346)) )/(2.( - 4.9))`

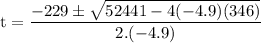
simplify multiplication:

`\rm\displaystyle t = ( - 229 \pm √( 52441- 6781.6) )/( - 9.8)`

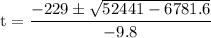
simplify Substraction:

`\rm\displaystyle t = ( - 229 \pm √( 45659.4) )/( - 9.8)`

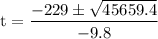
by simplifying we acquire:

`\displaystyle t = 48.2 \: \: \: \text{and} \quad - 1.5`

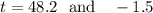
since time can't be negative

`\displaystyle t = 48.2`

hence,

at 48.2 seconds splashdown occurs

Question-2:

to figure out the maximum height we have to figure out the maximum Time first in that case the following formula can be considered

`\displaystyle x _{ \text{max}} = ( - b)/(2a)`

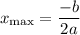
let a and b be -4.9 and 229 respectively thus substitute:

`\displaystyle t _{ \text{max}} = ( - 229)/(2( - 4.9))`

simplify which yields:

`\displaystyle t _{ \text{max}} = 23.4`

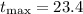
now plug in the maximum t to the function:

`\rm \displaystyle h(23.4)- 4.9 {(23.4)}^(2) + 229(23.4)+ 346`

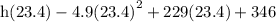
simplify:

`\rm \displaystyle h(23.4) = 3021.6`

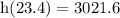
hence,

about 3021.6 meters high above sea-level the rocket gets at its peak?