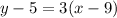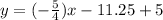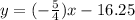Answer:
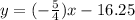
Explanation:
From the question we are told that:
Points of perpendicular bisector
(9,5),(−1,−3).
Generally the equation for slope is mathematically given by

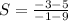
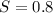
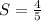
Therefore
Perpendicular lines of slope
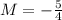
Generally the equation for perpendicular bisector Line is mathematically given by