Question

38. In a right triangle, if one acute angle has measure 8x + 5 and the other

has measure 4x + 9, find the larger of these two angles.
55.67
62.4
57.92
64.61
None of the above

Answer 1

`\huge\bold{Given :}`

Angle 1 = ( 8x + 5 ) ...(i)

Angle 2 = ( 4x + 9 ) ...(ii)

Angle 3 = 90° ( ∵ it is a right-angled triangle )

`\huge\bold{To\:find :}`

The value of the larger angle of the two acute angles.

`\large\mathfrak{{\pmb{\underline{\orange{Solution}}{\orange{:}}}}}`

`{\boxed{\mathcal{\red{ A. \:55.67° .\:}}}}`. ✅

`\large\mathfrak{{\pmb{\underline{\blue{Step-by-step\:explanation}}{\blue{:}}}}}`

We know that,

`\sf\pink{Sum\:of\:angles\:of\:a\:triangle\:=\:180°}`

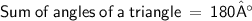
➪ ∠ 1 + ∠ 2 + ∠ 3 = 180°

➪ 8x + 5 + 4x + 9 + 90° = 180°

➪ 12x + 104° = 180°

➪ 12x = 180° - 104°

➪ 12x = 76°

➪ x =
`(76° )/(12)`

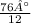
➪ x = 6.333°

The value of x is 6.333°.

Now,

Substituting the value of x in eq. (i) & (ii), we have

Angle 1 = 8x + 5

= 8 x 6.333 + 5

= 50.666 + 5

= 55.67°

Angle 2 = 4x + 9

= 4 x 6.333 + 9

= 25.332 + 9

= 34.332°

Clearly, angle 1 is greater than angle 2.

Therefore, the value of the larger angle of the two acute angles is 55.67°.

`\huge{\textbf{\textsf{{\orange{My}}{\blue{st}}{\pink{iq}}{\purple{ue}}{\red{35}}{\green{♡}}}}}`

Answer 2

We know that : The Sum of the angles in a triangle should be equal to 180°

Given : The Triangle is a Right angled Triangle

It means : One of the Angle in the triangle is 90°

It means : The sum of the other two angles should be equal to 90°

Given : One acute angle is 8x + 5 and the other one is 4x + 9

⇒ 8x + 5 + 4x + 9 = 90°

⇒ 12x + 14 = 90°

⇒ 12x = 76°

⇒ x = 6.333°

The larger angle among the acute angles is 8x + 5

⇒ 8(6.333) + 5

⇒ 50.67 + 5

⇒ 55.67