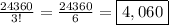Answer:
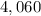
Explanation:
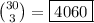
There are 30 teachers for the principal to choose from initially. After the principal chooses one, there will be 29, 28, 27, and so on.
Since the principal is only choosing 3, there are
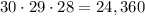 permutations. However, the order of which the principal chooses the teachers does not matter. In other words, without loss of generality, let the name of three of the teachers be Adam, Bernie, and Carla. Regardless of whether the principal chooses Adam then Bernie then Carla or Carla then Adam then Bernie, etcetera, the same three teachers are still being chosen.
permutations. However, the order of which the principal chooses the teachers does not matter. In other words, without loss of generality, let the name of three of the teachers be Adam, Bernie, and Carla. Regardless of whether the principal chooses Adam then Bernie then Carla or Carla then Adam then Bernie, etcetera, the same three teachers are still being chosen.
Thus, we must divide by the number of ways you can rearrange three distinct teachers, which is
 .
.
Therefore, the desired answer is