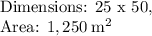Answer:
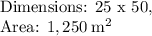
Explanation:
Let the one of the side lengths of the rectangle be
 and the other be
and the other be
 .
.
We can write the following equations, where
 will be the side opposite to the wall:
will be the side opposite to the wall:
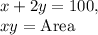
From the first equation, we can isolate
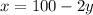 and substitute into the second equation:
and substitute into the second equation:
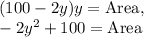
Therefore, the parabola
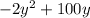 denotes the area of this rectangular enclosure. The maximum area possible will occur at the vertex of this parabola.
denotes the area of this rectangular enclosure. The maximum area possible will occur at the vertex of this parabola.
The x-coordinate of the vertex of a parabola in standard form
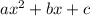 is given by
is given by
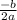 .
.
Therefore, the vertex is:
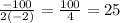
Plug in
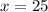 to the equation to get the y-coordinate:
to the equation to get the y-coordinate:

Thus the vertex of the parabola is at
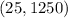 . This tells us the following:
. This tells us the following:
- The maximum area occurs when one side (y) of the rectangle is equal to 25
- The maximum area of the enclosure is 1,250 square meters
- The other dimension, from
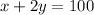 , must be
, must be
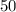
And therefore, the desired answers are: