Answer:
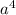 Where (a) is the side length of the hypercube
Where (a) is the side length of the hypercube
Explanation:
A dimension can be simply defined as a direction in which matter can move it.
We live in a 3-dimensional world, matter can move in three basic directions. The formula for finding the volume of a cube is the following: (
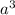 ). In the second dimension, a flat world, the area for the volume of a square is as follows: (
). In the second dimension, a flat world, the area for the volume of a square is as follows: (
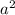 ). However, a hypercube is a paradox, as it exists in 4-dimensions, thus it cannot exist in our world. However, scientists propose "String theory" which requires that matter can move in more directs than the (3) we know of in our world. In essence, there can be more than 3 dimensions. A hypercube is the 4-dimensional version of a cube. If it follows the area pattern that a square-object follows in all preceding dimensions then the area of a hypercube is (
). However, a hypercube is a paradox, as it exists in 4-dimensions, thus it cannot exist in our world. However, scientists propose "String theory" which requires that matter can move in more directs than the (3) we know of in our world. In essence, there can be more than 3 dimensions. A hypercube is the 4-dimensional version of a cube. If it follows the area pattern that a square-object follows in all preceding dimensions then the area of a hypercube is (
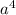 ).
).
Bear in mind that the area patter for a square-object is generally the following:
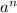
Where (a) is the side length of the square-object
(n) is the dimension the object is in.
Please keep in mind, this answer was written in the June of 2021, as science keeps progressing and new discoveries are made, this theory can be disproven. Therefore, this answer might be considered correct now, but it can be proven incorrect in the future.