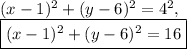Question

Determine the equation of the circle graphed below

Answer 1

(x-1)^2 + (y-6)^2 = 16

Explanation:

center = (1,6)

radius = 4

equation of a circle (x-h)^2+(y-k)^2=r^2

(x-1)^2 + (y-6)^2 = 16

Answer 2

`(x-1)^2+(y-6)^2=16`

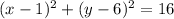
Explanation:

The equation of a circle is given by
`(x-h)^2+(y-k)^2=r^2`, where
`(h,k)` is the center of the circle and
`r` is the radius of the circle.

From the diagram, we can find the following:

• the radius of the circle is 4
• the center of the circle is located at (1,6)

Thus, we have:

`(x-1)^2+(y-6)^2=4^2,\\\boxed{(x-1)^2+(y-6)^2=16}`