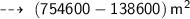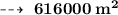Question

Find the area of the region between two concentric circular paths. If the radii of the circular paths are 210 m. and 490 m. respectively.​

Answer 1

Given :

• The radii of the circular paths are 210 m. and 490 m.

To Find :

• The area of the region between two concentric circular paths.

Solution :

Area of the outer circle :

`\: \qquad \dashrightarrow \sf{{ \pi \: * {(490)}^(2) {m}^(2)}}`

`\: \qquad \dashrightarrow \sf{{ \ (22)/(7) * \:490 * 490 \: {m}^(2)}}`

`\: \qquad \dashrightarrow \sf{{ \ \frac{22}{ \cancel{7}} * \cancel{490} * 490 \: {m}^(2)}}`

`\: \qquad \dashrightarrow \sf{{ \ {22} * 70 * 490 \: {m}^(2)}}`

`\: \qquad \dashrightarrow \bf{{ \ 754600 \: {m}^(2)}}`

⠀

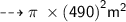
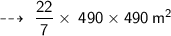
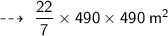
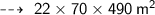
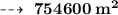
Area of the inner circle :

`\: \qquad \dashrightarrow \sf{{ \pi \: * {(210)}^(2) {m}^(2)}}`

`\: \qquad \dashrightarrow \sf{{ \ (22)/(7) * \:210 * 210 \: {m}^(2)}}`

`\: \qquad \dashrightarrow \sf{{ \ \frac{22}{ \cancel{7}} * \cancel{210} * 210 \: {m}^(2)}}`

`\: \qquad \dashrightarrow \sf{{ \ {22} * 30 * 210 \: {m}^(2)}}`

`\: \qquad \dashrightarrow \bf{{ \ 138600 \: {m}^(2)}}`

⠀

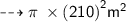
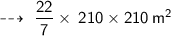
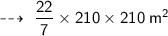
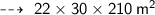
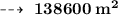
Therefore,

Area of the region inside the circular paths :

`\: \qquad \dashrightarrow \sf{{ \ (754600 - 138600 ) \: {m}^(2)}}`

`\: \qquad \dashrightarrow \bf{{ \ 616000 \: {m}^(2)}}`