Question

The faces on a number cube are labled 1,2,2,3,4, and 5the number cube is rolled 114 times how many times would you expect the number 2 to apper

Answer 1

Given:

The faces on a number cube are labeled 1,2,2,3,4, and 5.

The number cube is rolled 114 times.

To find:

How many times would you expect the number 2 to appear?

Solution:

We have,

Total outcomes = 1,2,2,3,4, and 5.

Number of total outcomes = 6

Favorable outcomes = 2 and 2

Number of favorable outcomes = 2

The probability of getting 2 is:

`P(2)=\frac{\text{Number of favorable outcomes}}{\text{Number of total outcomes}}`

`P(2)=(2)/(6)`

`P(2)=(1)/(3)`

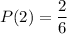
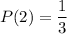
Now, the expected number of times when 2 to appear is:

`E(x)=114* P(2)`

`E(x)=114* (1)/(3)`

`E(x)=38`

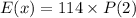
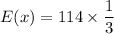
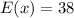
Therefore, the expected number of times is 38.