Explanation:
1.
Subtract the coefficient from both sides, keep 55 on the same side.
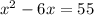
Complete the square by dividing the coefficient by two and squaring it.
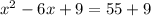
Use binomial to factor the left side.

2. Solve for x.
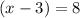
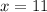
Remeber the square root of 64 is also -8 so
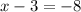

So the solutions are -5 and 11