Answer:
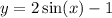
Explanation:
Let's break it down:
In the sinusoidal function
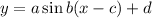 :
:
 represents Amplitude
represents Amplitude
 represents a constant related to the Period
represents a constant related to the Period
 represents Phase Shift
represents Phase Shift
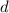 represents Vertical Shift
represents Vertical Shift
To find Amplitude, divide the entire height of the function (from top to bottom) by two, or find the vertical distance between the horizontal line of symmetry and the highest/lowest point of the wave. In this case, Amplitude is 2.
To find
 , use
, use
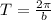 , where
, where
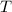 is the period of the function. To find the period, count the horizontal distance of the length of one complete cycle. In this case, period is
is the period of the function. To find the period, count the horizontal distance of the length of one complete cycle. In this case, period is
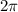 and therefore
and therefore
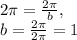 .
.
To find Phase Shift, find the horizontal shift from the parent function
 . In this case, there is no phase shift.
. In this case, there is no phase shift.
To find Vertical Shift, find the vertical shift from the parent function
 . In this case, vertical shift is -1.
. In this case, vertical shift is -1.
Thus, we've found:
Substituting these values into
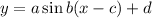 , we get:
, we get:
