Answer: (a) (i)50.42 m/s (ii) 51.35 m/s (iii) 52.094 m/s (iv) 52.2614 m/s (v) 52.27814 m/s
(b) 54.14 m/s
Explanation:
The average speed over the given time interval [a,b]: =
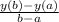
Given: If an arrow is shot upward on Mars with a speed of 56 m/s, its height in meters t seconds later is given by
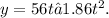
(a)
(i) average speed =
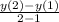
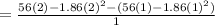
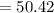 m/s
m/s
(ii) average speed =
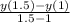
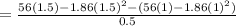
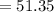 m/s
m/s
(iii) average speed =
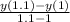
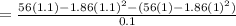
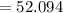 m/s
m/s
(iv) average speed =
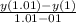

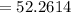 m/s
m/s
(v) average speed =
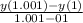

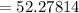 m/s
m/s
(b)
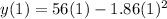

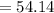 m/s
m/s