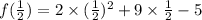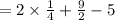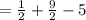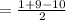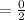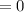Question

Find the zeros the polynomial fx=2x^2 +9x-5
pls help with steps ​

Answer 1

-5 ; 1/2

Explanation:

GIVEN :-

• A quadratic polynomial f(x) = 2x² + 9x - 5

TO FIND :-

• Zeroes of f(x) = 2x² + 9x - 5

GENERAL CONCEPTS TO BE USED IN THIS QUESTION :-

Lets say there's a quadratic polynomial f(x) = ax² + bx + c , whose factors are (x - α) & (x - β). To find the values of x for which f(x) will be zero , equate the factors of f(x) with 0.

⇒ (x - α) = 0 & (x - β) = 0

⇒ x = α & x = β

Hence, it can be concluded that if (x - α) & (x - β) are factors of f(x) , then α & β are the roots of f(x).

SOLUTION :-

Factorise f(x) = 2x² + 9x - 5.

• Split its middle term.

`=> 2x^2 + 10x - x - 5`

• Take '2x' common from first two terms & '-1' from last two terms.

`=> 2x(x + 5) - 1(x + 5)`

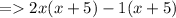
• Take (x + 5) common from the whole expression.

`=> (x + 5)(2x - 1)`

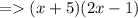
So , the factors of f(x) are (x + 5) & (2x - 1). Now equate the factors with zero.

⇒ (x + 5) = 0 & (2x - 1) = 0

⇒ x = -5 & x = 1/2

∴ The zeroes of f(x) = 2x² + 9x - 5 are (-5) & (1/2)

VERIFICATION :-

1) Put x = -5 in f(x) = 2x² + 9x - 5

⇒ f(-5) = 2(-5)² + 9×(-5) - 5

= 50 - 45 - 5

= 50 - 50

= 0

2) Put x = 1/2 in f(x) = 2x² + 9x - 5

`f((1)/(2) ) = 2 * ((1)/(2) )^2 + 9 * (1)/(2) - 5`

`= 2 * (1)/(4) + (9)/(2) - 5`

`= (1)/(2) + (9)/(2) - 5`

`= ( 1 + 9 - 10)/(2)`

`= (0)/(2)`

`= 0`