His question is incomplete, the complete question is;
A film oil on water will appear dark when it is very thin, because the path length difference becomes small compared with the wavelength of light and there is a phase shift at the top surface.
If the oil film becomes dark when the path length difference is less than one fourth the wavelength, what is the thickest the oil film can be and appear dark at all visible wavelengths. Oil has an index of fraction of 1.40.
In this question, assume the wavelength visible light is in the range of 400 nm to 700 nm.
Answer:
the minimum thickness of the oil for destructive interference to occur is approximately 35.714 nm
Step-by-step explanation:
Given the data in the question;
Path difference for destructive interference between the two reflected waves ( top and bottom );
δ = 2nt ---------- let this be equation 1
Also, for one path difference of reflected waves, one fourth of the wavelength of reflected waves;
δ = λ/4 ---------- let this be equation 2
from equation 1 and 2;
δ = δ
2nt = λ/4
we find t;
8nt = λ
t = λ / 8n
given that; λ
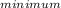 = 400 nm and n = 1.40
= 400 nm and n = 1.40
we substitute
t = 400nm / 8(1.40)
t = 400nm / 11.2
t = 35.7142857 ≈ 35.714 nm
Therefore, the minimum thickness of the oil for destructive interference to occur is approximately 35.714 nm