Answer:
(- 2, 3 ) and (
 ,
,
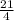 )
)
Explanation:
Given the 2 equations
y = 4x² + 8x + 3 → (1)
y = x + 5 → (2)
Substitute y = 4x² + 8x + 3 into (2)
4x² + 8x + 3 = x + 5 ( subtract x + 5 from both sides )
4x² + 7x - 2 = 0 ← in standard form
(x + 2)(4x - 1) = 0 ← in factored form
Equate each factor to zero and solve for x
x + 2 = 0 ⇒ x = - 2
4x - 1 = 0 ⇒ 4x = 1 ⇒ x =

Substitute these values into (2) for corresponding values of y
x = - 2 : y = - 2 + 5 = 3 ⇒ (- 2, 3 )
x =
 : y =
: y =
 + 5 =
+ 5 =
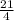 ⇒ (
⇒ (
 ,
,
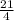 )
)