Question

HELP!!!! ASAP
NO LINKS!!!!

Answer 1

17.98

Explanation:

We can use the sum of a finite geometric series formula(in the picture). We can start by plugging in what we have. As of now, we have the first term, 12 and by dividing a term by a previous term, we can tell that the common ratio, r is 1/3. Lastly, we can say that n is equal to 6, because we are looking for the sum of the first 6 terms. After substituting we get:

`(12 - 12*((1)/(3))^6)/(1-(1)/(3) )`

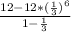
We can start by simplifying:

`(12-12((1)/(729)) )/((2)/(3) )`

`((2916)/(243) -(4)/(243) )/((2)/(3) )`

`((2912)/(243) )/((2)/(3) )`

`(2912)/(243)*(3)/(2)`

`(1456)/(81)`

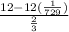
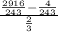
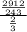
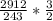
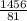
17.975308642

This can be rounded to 17.98

Answer 2

• 17.98

Explanation:

Given:

• a₁ = 12
• r = 1/3
• n = 6

Find the sum:

• Sₙ = 12(1 - (1/3)⁶)/(1 - 1/3) = 12(1 - 1/729) / (2/3) = 18(728/729) = 17.98 rounded