Answer:
x = 4
x = - 3
Explanation:
Method 1
Quadratic Formula
(3x + 9)(x - 4) = 0
3x(x - 4) + 9(x - 4) = 0
3x² - 12x + 9(x - 4) = 0
3x² - 12x + 9x - 36 = 0
3x² - 3x - 36 = 0
3(x² - x - 12) = 0
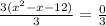
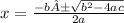
Ignore the A after the -b, wouldn't let me type it correctly. Ignore the A for the rest of the explanation as well.
x² - x - 12 = 0
a = 1
b = - 1
c = - 12
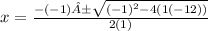
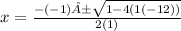
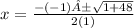
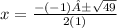
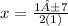

Separate into two equations.
One with addition and the other with subtraction.
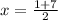
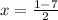
x = 4
x = - 3
Method 2
Factors
(3x + 9)(x - 4) = 0
3x(x - 4) + 9(x - 4) = 0
3x² - 12x + 9(x - 4) = 0
3x² - 12x + 9x - 36 = 0
3x² - 3x - 36 = 0
3(x² - x - 12) = 0
3(x² + 3x - 4x - 12) = 0
3(x² + 3x + (- 4x - 12)) = 0
3(x(x + 3) - 4(x + 3)) = 0
3(x - 4)(x + 3) = 0
Create separate equations
x - 4 = 0
x + 3 = 0
x - 4 = 0 ⇒ x - 4 + 4 = 0 + 4 ⇒ x = 4
x + 3 = 0 ⇒ x + 3 - 3 = 0 - 3 ⇒ x = - 3
x = 4
x = - 3