Question

Help please!

figure out the "inflection point" of the following function
f(x)=5x³ + 2x² − 3x​

Answer 1

`\displaystyle \left( - (2)/(15) , (286)/(675) \right)`

Explanation:

to figure out the infection point

take derivative both sides:

`\displaystyle f'(x) = (d)/(dx) {5x}^(3) + 2 {x}^(2) - 3x`

By sum derivation rule we acquire:

`\displaystyle \rm f'(x) = (d)/(dx) {5x}^(3) + (d)/(dx) 2 {x}^(2) - (d)/(dx) 3x`

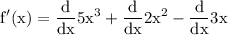
apply exponent derivation rule which yields:

`\displaystyle f'(x) = {15x}^(2) + 4{x}^{} - 3`

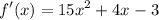
take derivative in both sides once again which yields:

`\rm\displaystyle f''(x) = (d)/(dx) {15x}^(2) + (d)/(dx) 4{x}^{} - (d)/(dx) 3`

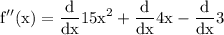
remember that, derivative of a constant is always 0 so,

`\rm\displaystyle f''(x) = (d)/(dx) {15x}^(2) + (d)/(dx) 4{x}^{} - 0`

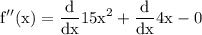
by exponent derivation rule we acquire:

`\rm\displaystyle f''(x) = {30x} + 4{}^{}`

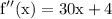
substitute f''(x) to 0 figure out the x coordinate of the inflection point:

`\rm\displaystyle {30x} + 4{}^{} = 0`

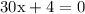
cancel 4 from both sides:

`\rm\displaystyle {30x} = - 4`

divide both sides by 30:

`\rm\displaystyle {x} = - (2)/(15)`

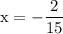
now plugin the value of x to the given function to figure out the y coordinate of the inflection point:

`\rm \displaystyle f(x) = {5 \left( - (2)/(15) \right) }^(3) + 2 {\left( - (2)/(15) \right) }^(2) - 3 \left( - (2)/(15) \right)`

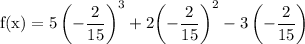
By simplifying we acquire:

`\displaystyle f(x) = (286)/(675)`

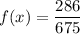
hence,

the coordinates of inflection point are

`\displaystyle \left( - (2)/(15) , (286)/(675) \right)`

Answer 2

x = -2/15.

Explanation:

Finding the second derivative:

f'(x) = 15x^2 +4x - 3

f"(x) = 30x + 4

30x + 4 = 0 for a point of inflection

x = -4/30 = -2/15

So 30x + 4 is negative up to x = -2/15 and positive thereafter.

So the curve passes from concave downward to concave upward at x = -2/15.