Question

Find the solution of the differential equation f' (t) = t^4+91-3/t

having the boundary condition f(1) =1/4

Answer 1

`\displaystyle f(t) = (t^5)/(5) + 91t - 3ln|t| - (1819)/(20)`

General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Equality Properties

• Multiplication Property of Equality
• Division Property of Equality
• Addition Property of Equality
• Subtraction Property of Equality

Algebra I

• Functions
• Function Notation

Calculus

Derivatives

Derivative Notation

Antiderivatives - Integrals

Integration Constant C

Integration Rule [Reverse Power Rule]:
`\displaystyle \int {x^n} \, dx = (x^(n + 1))/(n + 1) + C`

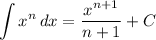
Integration Property [Addition/Subtraction]:
`\displaystyle \int {[f(x) \pm g(x)]} \, dx = \int {f(x)} \, dx \pm \int {g(x)} \, dx`

Integration Property [Multiplied Constant]:
`\displaystyle \int {cf(x)} \, dx = c \int {f(x)} \, dx`

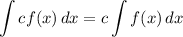
Explanation:

Step 1: Define

Identify

`\displaystyle f'(t) = t^4 + 91 - (3)/(t)`

`\displaystyle f(1) = (1)/(4)`

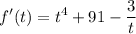
Step 2: Integration

Integrate the derivative to find function.

1. [Derivative] Integrate:
   `\displaystyle \int {f'(t)} \, dt = \int {t^4 + 91 - (3)/(t)} \, dt`
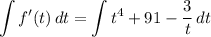
2. Simplify:
   `\displaystyle f(t) = \int {t^4 + 91 - (3)/(t)} \, dt`
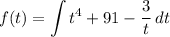
3. Rewrite [Integration Property - Addition/Subtraction]:
   `\displaystyle f(t) = \int {t^4} \, dt + \int {91} \, dt - \int {(3)/(t)} \, dt`
4. [1st Integral] Integrate [Integral Rule - Reverse Power Rule]:
   `\displaystyle f(t) = (t^5)/(5) + \int {91} \, dt - \int {(3)/(t)} \, dt`
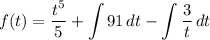
5. [2nd Integral] Integrate [Integral Rule - Reverse Power Rule]:
   `\displaystyle f(t) = (t^5)/(5) + 91t - \int {(3)/(t)} \, dt`
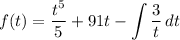
6. [3rd Integral] Rewrite [Integral Property - Multiplied Constant]:
   `\displaystyle f(t) = (t^5)/(5) + 91t - 3\int {(1)/(t)} \, dt`
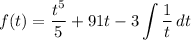
7. [3rd Integral] Integrate:
   `\displaystyle f(t) = (t^5)/(5) + 91t - 3ln|t| + C`

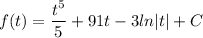
Our general solution is
`\displaystyle f(t) = (t^5)/(5) + 91t - 3ln|t| + C`.

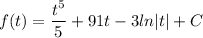Step 3: Find Particular Solution

Find Integration Constant C for function using given condition.

1. Substitute in condition [Function]:
   `\displaystyle f(1) = (1^5)/(5) + 91(1) - 3ln|1| + C`
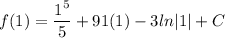
2. Substitute in function value:
   `\displaystyle (1)/(4) = (1^5)/(5) + 91(1) - 3ln|1| + C`
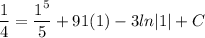
3. Evaluate exponents:
   `\displaystyle (1)/(4) = (1)/(5) + 91(1) - 3ln|1| + C`
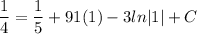
4. Evaluate natural log:
   `\displaystyle (1)/(4) = (1)/(5) + 91(1) - 3(0) + C`
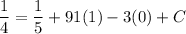
5. Multiply:
   `\displaystyle (1)/(4) = (1)/(5) + 91 - 0 + C`
6. Add:
   `\displaystyle (1)/(4) = (456)/(5) - 0 + C`
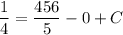
7. Simplify:
   `\displaystyle (1)/(4) = (456)/(5) + C`
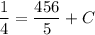
8. [Subtraction Property of Equality] Isolate C:
   `\displaystyle -(1819)/(20) = C`
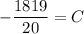
9. Rewrite:
   `\displaystyle C = -(1819)/(20)`
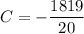
10. Substitute in C [Function]:
    `\displaystyle f(t) = (t^5)/(5) + 91t - 3ln|t| - (1819)/(20)`

∴ Our particular solution to the differential equation is
`\displaystyle f(t) = (t^5)/(5) + 91t - 3ln|t| - (1819)/(20)`.

Topic: AP Calculus AB/BC (Calculus I/II)

Unit: Integration

Book: College Calculus 10e