Answer:
See below for answers and explanations
Explanation:
Part A:
Given:
Pooled sample size:

Sample size (with academic scholarships):

Sample size (no academic scholarships):
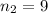
Population standard deviations: Unknown
Sample mean (with academic scholarships):
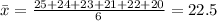
Sample mean (no academic scholarship):
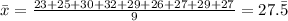
Sample standard deviation (with academic scholarships):

Sample standard deviation (no academic scholarships):
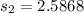
Degrees of freedom:
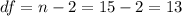
Significance level:

Decide which test is most appropriate to conduct:
Therefore, we will conduct a 2-sample t-test assuming our conditions are satisfied.
List null and alternate hypotheses:
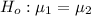 -> There's no difference in ACT scores between students with and without an academic scholarship
-> There's no difference in ACT scores between students with and without an academic scholarship
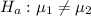 -> There's a difference in ACT scores between students with and without an academic scholarship (it's two-sided)
-> There's a difference in ACT scores between students with and without an academic scholarship (it's two-sided)
Determine the value of the test statistic:
We will use the formula
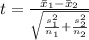 to compute the test statistic
to compute the test statistic
 . Therefore, the test statistic is
. Therefore, the test statistic is
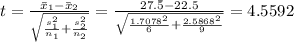
Calculate the p-value:
Because the test is two-sided,
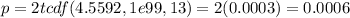
Interpret p-value and conclude test:
Given our significance level is
 , since
, since
 , we reject the null hypothesis and conclude that there is significant evidence that suggests that there is a difference in ACT scores between students with and without an academic scholarship (it's more likely that the alternate hypothesis is true)
, we reject the null hypothesis and conclude that there is significant evidence that suggests that there is a difference in ACT scores between students with and without an academic scholarship (it's more likely that the alternate hypothesis is true)
Part B:
The formula for a confidence interval for the difference in 2 population means is
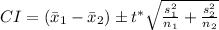 where
where
 is the difference of the 2 sample means and
is the difference of the 2 sample means and
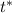 is the critical score for the desired confidence level.
is the critical score for the desired confidence level.
The critical score for our 98% confidence interval would be
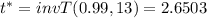
Therefore, our 98% confidence interval for the difference in the ACT scores between students with and without an academic scholarship is
![CI=(27.\bar{5}-22)\pm 2.6503\sqrt{(1.7078^2)/(6)+(2.5868^2)/(9)}}=[2.6167,8.4944]](https://img.qammunity.org/2022/formulas/mathematics/high-school/ff0yfgj2fendd9ng9mbkcht4oy9gk3bu0o.png)
This means that we are 98% confident that the true difference in the ACT scores between students with and without an academic scholarship is contained within the interval
![[2.6167,8.4944]](https://img.qammunity.org/2022/formulas/mathematics/high-school/lqbb7ix11vr76zfbe42xcnuwzksilpgdsg.png)