Question

Suppose a sample of 80 with a sample proportion of 0.58 is taken from a

population. Which of the following is the approximate 99.7% confidence
interval for the population parameter?

Answer 1

(0.414, 0.746)

Explanation:

big brain

Answer 2

99.7% confidence interval is
`[0.4162,0.7437]`

Explanation:

The formula for a confidence interval for a population proportion is
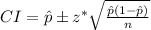`CI=\hat{p}\pm z^*\sqrt{\frac{\hat{p}(1-\hat{p})}{n} }` where
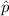`\hat{p}` is the sample proportion,
`n` is the sample size, and
`z^*` is the critical score for the desired confidence level.

We are given a sample size of
`n=80` and a sample proportion of
`\hat{p}=0.58`. Our critical score for a 99.7% confidence level would be
`z^*=normalcdf(0.9985,0,1)=2.9677`

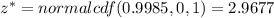
Therefore, the approximate 99.7% confidence interval for the population parameter is
`CI=\hat{p}\pm z^*\sqrt{\frac{\hat{p}(1-\hat{p})}{n} }=0.58\pm 2.9677\sqrt{(0.58(1-0.58))/(80) }=[0.4162,0.7438]`

So we are 99.7% confident that the true population proportion is contained within the interval
`[0.4162,0.7437]`