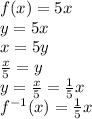Answer:
f^(-1)(x) = 1/5x
Explanation:

Inverse Function is when we swap the x-term and y-term. Here is an explanation to the inverse of linear function above.
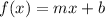
Here we define f(x) = y. It is better to use y instead of f(x)
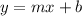
Swap x-term and y-term.
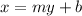
Arrange/Simplify in the y-isolated equation.

Since the function is in inverse form. It's recommended to use f^(-1) to point out that the function is an inverse of the original function.
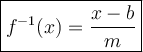
Hence, y = (x-b)/m is an inverse of y = mx+b. A one-to-one function can have an inverse form. Back to the question!
We are given the linear function:
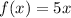
We will be using substitution method by substituting the original function in inverse form.
Since we know that the slope is 5 and doesn't have y-intercept which is b-value. Hence

Or we swap x-term and y-term.