Question

How can I do this ???​

Answer 1

The triangle EFG can be constructed with the following specifications:
`EF = 8\,cm`,
`FG = 7\,cm`,
`EG = 12\,cm`,
`E \approx 34.093^(\circ)`,
`F \approx 106.068^(\circ)`,
`G \approx 39.838^(\circ)`.

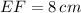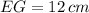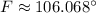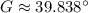Explanation:

A triangle is formed by either knowing the lengths of its three sides or knowing two angles and the length of a side or knowing a angle and the lengths of two sides. By Geometry, we know that sum of internal angles in triangles equals 180°. In order to construct this triangle, we need to know the measures of angles E, F and G by means of the Law of Cosine:

Angle E

`E = \cos^(-1)\left[((7\,cm)^(2)-(12\,cm)^(2)-(8\,cm)^(2))/(-2\cdot (12\,cm)\cdot (8\,cm)) \right]`

`E \approx 34.093^(\circ)`

Angle F

`F = \cos^(-1)\left[((12\,cm)^(2) - (8\,cm)^(2) - (7\,cm)^(2))/(-2\cdot (8\,cm)\cdot (7\,cm)) \right]`

`F \approx 106.068^(\circ)`

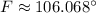
Angle G

`G = \cos^(-1)\left[((8\,cm)^(2)-(12\,cm)^(2)-(7\,cm)^(2))/(-2\cdot (12\,cm)\cdot (7\,cm)) \right]`

`G \approx 39.838^(\circ)`

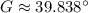
The triangle EFG can be constructed with the following specifications:
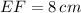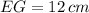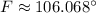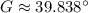`EF = 8\,cm`,
`FG = 7\,cm`,
`EG = 12\,cm`,
`E \approx 34.093^(\circ)`,
`F \approx 106.068^(\circ)`,
`G \approx 39.838^(\circ)`.