Question

Find the Area of the figure below, composed of a rectangle with a semicircle

removed from it. Round to the nearest tenths place.

Answer 1

Area of the figure = 25.7 units²

Explanation:

Area of the given figure = Area of rectangle ABCD - Area of the semicircle with diameter CD

Area of the rectangle ABCD = Length × Width

= BC × AB

= 8 × 4

= 32 units²

Area of the semicircle =
`(1)/(2)\pi r^(2)`

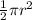
Here, r = radius of the semicircle

r =
`\frac{\text{Diameter}}{2}`

r =
`(1)/(2)(CD)`

r =
`(4)/(2)`

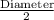
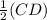
r = 2 units

Therefore, area of the semicircle =
`(1)/(2)\pi (2)^2`

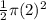
= 2π

= 6.28 units²

Area of the given figure = 32 - 6.28

= 25.72 units²

≈ 25.7 units²