Answer:
D. Yes, since the p-value of the one-sided test is less than 5%.
Explanation:
Test if there is evidence that the proportion of large trucks carrying hazardous materials is less than 30%.
At the null hypothesis, we test that this proportion is of 30%, that is:
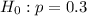
At the alternate hypothesis, we test if this proportion is less than 30%, that is:

The test statistic is:

In which X is the sample mean,
 is the value tested at the null hypothesis,
is the value tested at the null hypothesis,
 is the standard deviation and n is the size of the sample.
is the standard deviation and n is the size of the sample.
0.3 is tested at the null hypothesis:
This means that
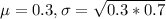
Of 350 randomly selected trucks, 87 were carrying hazardous materials.
This means that
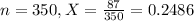
Value of the test statistic:



P-value of the test and decision:
The p-value of the test is the probability of finding a sample proportion below 0.2486, which is the p-value of z = -2.1.
Looking at the z-table, z = -2.1 has a p-value of 0.0179.
The p-value of the test is 0.0179 < 0.05, which means that there is evidence at the 5% level that the proportion of large trucks carrying hazardous materials is less than 30%. Thus, the correct answer is given by option D.