Answer:
The statements that complete the proofs are;
First statement
One half the measure of their intercepted arcs
Second statement
mARC CDE = 2·m∠CFE and mARC CFE = 2·m∠CDE
Explanation:
The two column proof that ∠CFE and ∠CDE are supplementary is presented as follows;
Statement
 Reason
Reason
Quadrilateral CDEF is inscribed is circle A
 Given
Given
 +
+
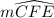 = 360°
= 360°
 Measure of angle round a circle
Measure of angle round a circle
∠CFE and ∠CDE are inscribed angles
 Given
Given
∠CFE + ∠CDE = 1/2 × (
 +
+
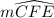 )
)
 Inscribed angles are (i) one half the measure of their intercepted arcs
Inscribed angles are (i) one half the measure of their intercepted arcs
2 × (∠CFE + ∠CDE) = (
 +
+
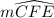 )
)
So, (ii)
 = 2 × m∠CFE and
= 2 × m∠CFE and
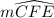 = 2 × m∠CDE From the inscribed angle theorem above (See attached drawing)
= 2 × m∠CDE From the inscribed angle theorem above (See attached drawing)
2·m∠CFE + 2·m∠CDE = 360°
 Using substitution property of equality
Using substitution property of equality
(2·m∠CFE + 2·m∠CDE)/2 = 360°/2 → m∠CFE + m∠CDE) = 180°
 Dividing both sides by 2
Dividing both sides by 2
m∠CFE and m∠CDE) are supplementary
 Angles that sum up to 180°
Angles that sum up to 180°
The statements that complete the proofs are;
(i) One half the measure of their intercepted arcs
(ii) mARC CDE = 2·m∠CFE and mARC CFE = 2·m∠CDE