Question

10. In a survey of 212 people at the local track and field championship, 72% favored the home team

winning
a. Find the margin of error for the survey.
b. Give the 95% confidence interval that is likely to contain the exact percent of all people who
favor the home team winning.

Answer 1

The margin of error for the survey is 0.0308 . The 95% confidence interval is (0.6596 ; 0.7804).

Using the parameters given for our Calculation;

• p = 72% = 0.72
• n = 212

The margin of error is defined thus :

• √p(1 - p)/n

Now we have :

Margin of Error = √0.72(1 - 0.72)/212

=√0.72(0.18)/212

= √0.2016/212

= √0.0009509

= 0.0308

The confidence Interval is defined thus :

• p ± (Zcritical * margin of error)
• Zcritical at 95% confidence level = 1.96

Now we have :

0.72 ± (1.96 × 0.0308)

0.72 ± 0.0604

The confidence interval (0.72 - 0.0604 ; 0.72 + 0.0604)

Therefore, the confidence interval is 0.6596 ; 0.7804

Answer 2

a. The margin of error for the survey is of 0.0308 = 3.08%.

b. The 95% confidence interval that is likely to contain the exact percent of all people who favor the home team winning is (65.96%, 78.04%).

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the z-score that has a p-value of
`1 - (\alpha)/(2)`.

The margin of error of the survey is:

`M = \sqrt{(\pi(1-\pi))/(n)}`

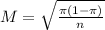
The confidence interval can be written as:

`\pi \pm zM`

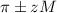
In a survey of 212 people at the local track and field championship, 72% favored the home team winning.

This means that
`n = 212, \pi = 0.72`

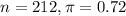
a. Find the margin of error for the survey.

`M = \sqrt{(0.72*0.28)/(212)} = 0.0308`

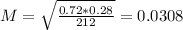
The margin of error for the survey is of 0.0308 = 3.08%.

b. Give the 95% confidence interval that is likely to contain the exact percent of all people who favor the home team winning.

95% confidence level

So
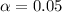`\alpha = 0.05`, z is the value of Z that has a p-value of
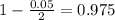`1 - (0.05)/(2) = 0.975`, so
`Z = 1.96`.

Lower bound:

`\pi - zM = 0.72 - 1.96*0.0308 = 0.6596`

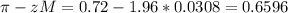
Upper bound:

`\pi + zM = 0.72 + 1.96*0.0308 = 0.7804`

As percent:

0.6596*100% = 65.96%

0.7804*100% = 78.04%.

The 95% confidence interval that is likely to contain the exact percent of all people who favor the home team winning is (65.96%, 78.04%).