Answer:
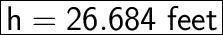
Explanation:
See the attached file along with the solution.
Let the height be h,
base of triangle 1 be x
base of triangle 2 be 100 - x
Using the following trigonometry for both the triangles.
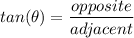
For triangle 1:
tan 20 = h / x ---------------------(1)
For triangle 2:
tan 45 = h / 100 - x -----------------(2)
Taking Eq. (1)
tan 20 = h / x
Multiply both sides by x
tan 20 * x = h
h = tan (20) * x
h = 0.36397x -----------------------(3)
Put Eq. (3) in (2)
tan (45) = 0.36397x / 100-x
1 = 0.36397 x / 100 - x
Multiply both sides by 100 - x
100 - x = 0.36397 x
Add x to both sides
100 = 0.36397 x + x
100 = 1.36397 x
Divide both sides by 1.36397
100 / 1.36397 = x
73.315 = x
x = 73.315 feet
Now, Finding h (height):
tan 20 = h / x (Eq. (1))
h = tan (20) * x
h = 0.36397 * 73.315
h = 26.684 feet
![\rule[225]{225}{2}](https://img.qammunity.org/2022/formulas/mathematics/high-school/3icqlwn6du2l5ygbr7z2lp6sjjralcpq09.png)
Hope this helped!
~AH1807