Question

If a system has cind independent components, what is the maximum number of phases that can exist in equilibrium? (b) A given system has eight liquid phases in equilibrium with each other. What must be true about the number of independent components in this system?

Answer 1

a) the maximum number of phases that can exist in equilibrium is
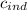`c_{ind` + 2

b) the number of independent components in this system are 6

Step-by-step explanation:

We know that, The degree of freedom f for a system can be simply referred to as number of variables that must be defined to completely solve a system.

If degree of freedom is 0, then any problem is can be solved.

a) If a system has cind independent components, what is the maximum number of phases that can exist in equilibrium?

The degree of freedom for a system can be written as;

f =
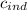`c_{ind` - p + 2

where f is the degree of freedom

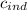`c_{ind` is the number of independent component

so we solve for
`c_{ind`

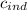
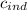`c_{ind` = f + p - 2

we know that f can not be less than 0

Hence maximum possible face will be;

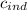`c_{ind` = p - 2

p =
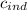`c_{ind` + 2

Therefore, the maximum number of phases that can exist in equilibrium is
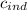`c_{ind` + 2

b) A given system has eight liquid phases in equilibrium with each other. What must be true about the number of independent components in this system?

Number of phases in the system p = 8

p =
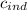`c_{ind` + 2

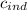`c_{ind` = p - 2

we substitute

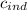`c_{ind` = 8 - 2

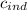`c_{ind` = 6

Therefore, the number of independent components in this system are 6