Answer:
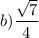
Explanation:
we are given that

we want to figure out Cos

in order to do so we can consider Pythagoras trig indentity given by
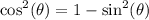
given that,sin
 =¾
=¾
thus substitute,

simplify square:
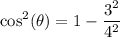
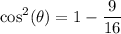
simplify substraction:
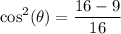
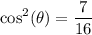
square root both sides:
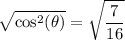
By square root property:
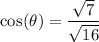
simplify root:

hence, our answer is b