Question

I wander what the quadramatic fourmula is

Answer 1

Definition of quadratic a formula
= a formula that gives the solutions of the general quadratic equation ax2 + bx + c = 0 and that is usually written in the form x = (-b ± √(b2 − 4ac))/(2a)

Answer 2

QUADRATIC FORMULA

The quadratic formula is a formula that offers the solution(s) to a quadratic equation in elementary algebra. Factoring (direct factoring, grouping, AC technique), completing the square, graphing, and other methods can be used instead of the quadratic formula to solve a quadratic problem.

Given the form of a generic quadratic equation

ax² + bx + c = 0

The quadratic formula is: whose discriminant b2 - 4ac is positive (with x representing an unknown, a, b, and c representing constants with a 0).

`\rm{ \green{x _(1) = \frac{ - b \frac{ + }{} \sqrt{b {}^(2) - 4ac } }{2a} }}`

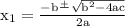
The plus–minus symbol "" denotes that the quadratic equation has two solutions, which are written separately as:

`\rm{ \green{x _(1) \frac{ - b + \sqrt{b {}^(2) - 4ac } }{2a} }} \: and \: \rm{ \green{x _(2) \frac{ - b - \sqrt{b {}^(2) - 4ac } }{2a} }}`

Each of these two solutions is referred to as a quadratic equation root. These roots show the x-values at which any parabola, expressed explicitly as y = ax2 + bx + c, crosses the x-axis geometrically.

The quadratic formula, in addition to yielding the zeros of any parabola, can also be used to determine the parabola's axis of symmetry and the number of real zeros in the quadratic equation.