Question

Is (x + 2) a factor of x + 6x2 + 3x – 10?

Answer 1

Assuming that you actually mean that if
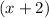`(x+2)` is a factor of
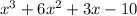`x^(3)+6x^(2)+3x-10`, the answer is yes.

Explanation:

We need to do long division of the polynomial to figure out if this is true. I would show it here, but it is extremely difficult to do so. (Maybe someone could help me.) When I factor it, it does not leave a remainder, so this is true.

`x^(3)+6x^(2)+3x-10= (x+2)(x^2+4x-5)`

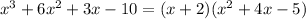
Another way one could figure this out is by graphing the equation
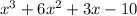`x^(3)+6x^(2)+3x-10` on your graphing calculator or on just a graphing website, lake Desmos. The screenshot below shows the graph.

The polynomial will have a factor of
`(x-c)` if the point
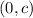`(0,c)` exists on the graph. The graph has the following three zeroes.

`(0,-2)`,
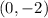`(0, -5)`, and
`(0,1)`

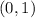
As such, it has the three factors
`(x-(-2))`,
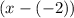`(x-(-5))`, and
`(x-1)`.

Since
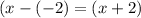`(x-(-2)=(x+2)`, it is a factor of the polynomial.

Answer 2

no

Explanation:

6x² + 4x - 10 in factored form is:

2(3x+5)(x-1)