Question

he wait time (after a scheduled arrival time) in minutes for a train to arrive is Uniformly distributed over the interval [0,15]. You observe the wait time for the next 95 trains to arrive. Assume wait times are independent. Part a) What is the approximate probability (to 2 decimal places) that the sum of the 95 wait times you observed is between 670 and 796

Answer 1

81.74% probability that the sum of the 95 wait times you observed is between 670 and 796

Explanation:

To solve this question, the uniform probability distribution and the normal probability distribution must be understood.

Uniform distribution:

A distribution is called uniform if each outcome has the same probability of happening.

The distribution has two bounds, a and b.

Its mean is given by:

`M = (b - a)/(2)`

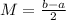
Its standard deviation is given by:

`S = \sqrt{((b-a)^2)/(12)}`

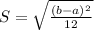
Normal distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

n instances of the uniform distribution can be approximated to the normal with
`\mu = nM`,
`\sigma = S√(n)`

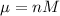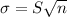
Uniformly distributed over the interval [0,15].

This means that:

`M = (15 - 0)/(2) = 7.5`

`S = \sqrt{((15-0)^2)/(12)} = 4.33`

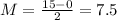
95 trains

`n = 95`, so:

`\mu = 95M = 95*7.5 = 712.5`

`\sigma = S√(n) = 4.33√(95) = 42.2`

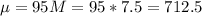
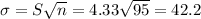
What is the approximate probability (to 2 decimal places) that the sum of the 95 wait times you observed is between 670 and 796?

This is the pvalue of Z when X = 796 subtracted by the pvalue of Z when X = 670. So

X = 796

`Z = (X - \mu)/(\sigma)`

`Z = (796 - 712.5)/(42.2)`

`Z = 1.98`

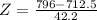
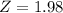
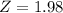`Z = 1.98` has a pvalue of 0.9761

X = 670

`Z = (X - \mu)/(\sigma)`

`Z = (670 - 712.5)/(42.2)`

`Z = -1`

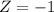
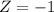`Z = -1` has a pvalue of 0.1587

0.9761 - 0.1587 = 0.8174

81.74% probability that the sum of the 95 wait times you observed is between 670 and 796