Question

The moon Ariel orbits Uranus at a distance of 1.91 x 108 m once every 2.52 days. Use that data to calculate the mass of Uranus. [?] x 10kg​

Answer 1

Mu = 8.66 × 10^25 kg

Step-by-step explanation:

centripetal force = gravitational force

`m \frac{ {v}^(2) }{r} = (grav.const) \frac{m * mu}{ {r}^(2) }`

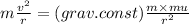
where

m = mass of moon Ariel

mu = mass of Uranus

r = radius of Ariel's orbit

v = Ariel's velocity around Uranus

To find the velocity, we need to find the circumference of the no orbit and then divide it by the period (2.52 days):

circumference = 2πr = 2π×(1.91 × 10^8 m)

= 1.2 × 10^9 m

period = 2.52 days × (24 h/1 day)×(3600 s/1 hr)

= 2.18 × 10^5 s

v = (1.2 × 10^9 m)/(2.18 × 10^5 s)

= 5.5 × 10^3 m/s

(5.5 × 10^3 m/s)^2/(1.91 × 10^8 m) = (6.67 × 10^-11 m^3/kg-s^2)Mu/(1.91 × 10^8 m)^2

Solving Mu,

Mu = 8.66 × 10^25 kg