Question

Find the P-value for a test of the claim that more than 60% of the people following a particular diet will experience increased energy (H1: p > 0.6). Of 100 randomly selected subjects who followed the diet, 70 noticed an increase in their energy level. 2.041 1.5486 1.2743 2.536

Answer 1

The p-value of the test is 0.0207.

Explanation:

Test of the claim that more than 60% of the people following a particular diet will experience increased energy (H1: p > 0.6).

The null hypothesis is:

`H_0: p = 0.6`

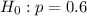
The alternate hypothesis is:

`H_1: p > 0.6`

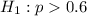
The test statistic is:

`z = (X - \mu)/((\sigma)/(√(n)))`

In which X is the sample mean,
`\mu` is the value tested at the null hypothesis,
`\sigma` is the standard deviation and n is the size of the sample.

0.6 is tested at the null hypothesis:

This means that
`\mu = 0.6, \sigma = √(0.6*0.4)`

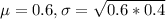
Of 100 randomly selected subjects who followed the diet, 70 noticed an increase in their energy level.

This means that
`n = 100, X = (70)/(100) = 0.7`

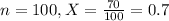
Test statistic:

`z = (X - \mu)/((\sigma)/(√(n)))`

`z = \frac{0.7 - 0.6}{\frac{\\sqrt{0.6*0.4}}{√(100)}}`

`z = 2.04`

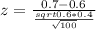
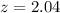
P-value of the test:

The p-value of the test is the probability of finding a sample proportion of at least 0.7, which is 1 subtracted by the p-value of z = 2.04.

Looking at the z-table, z = 2.04 has a p-value of 0.9793

1 - 0.9793 = 0.0207

The p-value of the test is 0.0207.