Given:
The equation of the parabola is:
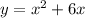
To find:
The y-intercept of the parabola.
Solution:
We have,
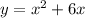
Putting
 in the given equation, we get
in the given equation, we get
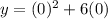

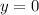
Therefore, the y-intercept of the parabola is 0. It means the y-intercept of the given parabola is at point (0,0).