Answer:
144°
Explanation:
First, find the area of the circle, with the formula A =
 r²
r²
Plug in 10 as the radius, and solve
A =
 r²
r²
A =
 (10²)
(10²)
A = 100

Using this, create a proportion that relates the area of the sector to the degree measure of the arc.
Let x represent the degree measure of the arc of the sector:
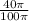 =
=

Cross multiply and solve for x:
100
 x = 14400
x = 14400

x = 144
So, the degree measure of the sector arc is 144°