Answer:
a) -6 mpg.
b) 2.77 mpg
c) The 95% confidence interval for the difference of population mean gas mileages for engines A and B and interpret the results, in mpg, is (-8.77, -3.23).
Explanation:
To solve this question, we need to understand the central limit theorem, and subtraction of normal variables.
Central Limit Theorem
The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
 and standard deviation
and standard deviation
 , the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
 and standard deviation
and standard deviation
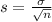 .
.
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
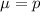 and standard deviation
and standard deviation
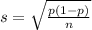
Subtraction between normal variables:
When two normal variables are subtracted, the mean is the difference of the means, while the standard deviation is the square root of the sum of the variances.
Gas mileage A: Mean 36, standard deviation 6, sample of 50:
So
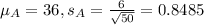
Gas mileage B: Mean 42, standard deviation 8, sample of 50:
So
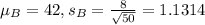
Distribution of the difference:
Mean:
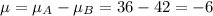
Standard error:
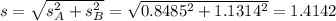
A. Find the point estimate.
This is the difference of means, that is, -6 mpg.
B. Find the margin of error
We have that to find our
 level, that is the subtraction of 1 by the confidence interval divided by 2. So:
level, that is the subtraction of 1 by the confidence interval divided by 2. So:
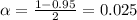
Now, we have to find z in the Ztable as such z has a pvalue of
 .
.
That is z with a pvalue of
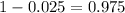 , so Z = 1.96.
, so Z = 1.96.
Now, find the margin of error M as such
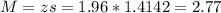
The margin of error is of 2.77 mpg
C. Construct the 95% confidence interval for the difference of population mean gas mileages for engines A and B and interpret the results(5 pts)
The lower end of the interval is the sample mean subtracted by M. So it is -6 - 2.77 = -8.77 mpg
The upper end of the interval is the sample mean added to M. So it is -6 + 2.77 = -3.23 mpg
The 95% confidence interval for the difference of population mean gas mileages for engines A and B and interpret the results, in mpg, is (-8.77, -3.23).