Given:
The equation of the trend line is:
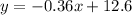
In this equation y represents the wind chill and x represents the wind speed.
To find:
The wind chill at 51 mi/h.
Solution:
We have,
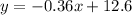
Putting
 , we get
, we get
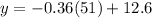


Round the answer to the nearest degree.
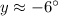
Therefore, the wind chill at wind speed 51 mi/h is -6°.